The state diagram of a system is shown below. A system is described by the state-variable equations $$\dot{X}= AX+Bu;\:\: y = CX+Du$$
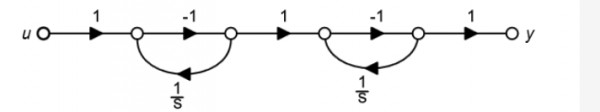
The state transition matrix $e^{At}$ of the system shown in the figure above is
- $\begin{bmatrix} e^{-t}& 0\\te^{-t} &e^{-t} \end{bmatrix}$
- $\begin{bmatrix} e^{-t}& 0\\-te^{-t} &e^{-t} \end{bmatrix}$
- $\begin{bmatrix} e^{-t}& 0\\e^{-t} &e^{-t} \end{bmatrix}$
- $\begin{bmatrix} e^{-t}&-te^{-t} \\ 0 &e^{-t} \end{bmatrix}$