GATE ECE 2021 | GA Question: 10

Question

​​

Corners are cut from an equilateral triangle to produce a regular convex hexagon as shown in the figure above.

The ratio of the area of the regular convex hexagon to the area of the original equilateral triangle is

• A. $2:3$
• B. $3:4$
• C. $4:5$
• D. $5:6$

Answer 1

Given that, corners are cut from an equilateral triangle to produce a regular convex hexagon. The smaller triangles cut are equilateral triangles (If we cut off corners to create a regular hexagon, then each angle of the hexagon is $120^{\circ}$, and so each angle of every removed triangle is $60^{\circ}$ making these triangles equilateral)

Let the original triangle side be $3a.$

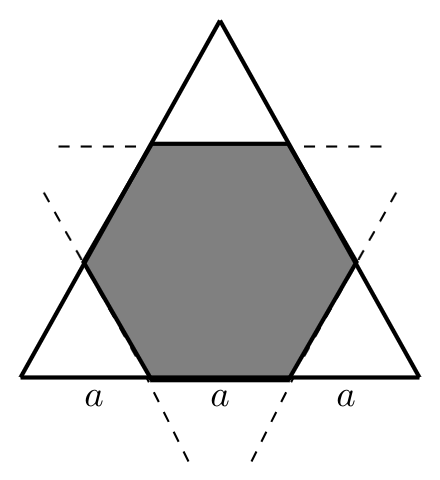
The area of regular convex hexagon $H_{1} = \frac{3\sqrt{3}}{2}s^{2},$ the area of equilateral triangle $ T_{1}= \frac{\sqrt{3}}{4}s^{2},$ where $s$ is the side length. 

Now, $H_{1} = \frac{3\sqrt{3}}{2}a^{2},A_{1} = \frac{\sqrt{3}}{4}(3a)^{2} = \frac{9\sqrt{3}}{4}a^{2}$

The required ratio $ = \dfrac{H_{1}}{A_{1}} = \dfrac{\frac{3\sqrt{3}}{2}a^{2}}{\frac{9\sqrt{3}}{4}a^{2}} = \frac{2}{3}.$

$\therefore$ The ratio of the area of the regular convex hexagon to the area of the original equilateral triangle is $2:3.$

So, the correct answer is $(A).$

Comments

How's it proved that the smaller triangles are equilateral?

---

If we cut off corners to create a regular hexagon, then each angle of the hexagon is $120^{\circ}$, meaning that each angle of each removed triangle is $60^{\circ}$, so these triangles are equilateral.

From here:https://math.stackexchange.com/questions/3726183/corners-are-cut-off-from-an-equilateral-triangle-to-produce-a-regular-hexagon-a

---

We can also see that the pink triangles are also equilateral and of equal area therefore we can directly count the number of triangles in whole triangle and hexagon to find the ratio of area. 

$$\frac{\text{pink triangles}}{\text{total triangles}} = \frac{6}{9} $$