For the signal flow graph shown in the figure, the value of $\dfrac{C(s)}{R(s)}$ is
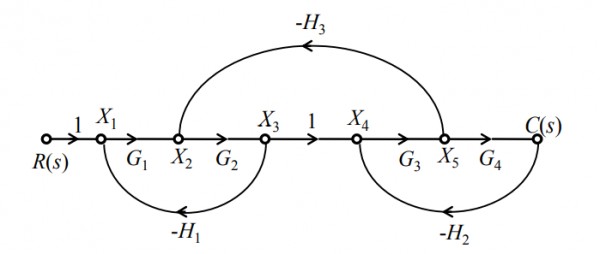
- $\dfrac{G_{1}G_{2}G_{3}G_{4}}{1 – G_{1}G_{2}H_{1} – G_{3}G_{4}H_{2} – G_{2}G_{3}H_{3} +G_{1}G_{2}G_{3}G_{4}H_{1}H_{2}} \\$
- $\dfrac{G_{1}G_{2}G_{3}G_{4}}{1 + G_{1}G_{2}H_{1} + G_{3}G_{4}H_{2} + G_{2}G_{3}H_{3} +G_{1}G_{2}G_{3}G_{4}H_{1}H_{2}} \\$
- $\dfrac{1}{1 + G_{1}G_{2}H_{1} + G_{3}G_{4}H_{2} + G_{2}G_{3}H_{3} +G_{1}G_{2}G_{3}G_{4}H_{1}H_{2}} \\$
- $\dfrac{1}{1 – G_{1}G_{2}H_{1} – G_{3}G_{4}H_{2} – G_{2}G_{3}H_{3} +G_{1}G_{2}G_{3}G_{4}H_{1}H_{2}}$